setrvip.blogg.se - Quadratic inequalities

Quadratic inequalities how to#

Solving Equations by Multiplication, Division, or Taking the Reciprocal.Solving Equations by Addition or Subtraction.

Quadratic inequalities how to#

The topic ends with information on how to solve equations that require multiple steps and equations that include multiple variables. It starts with the basic concepts that will be used, including isolating the variable and combining like terms, before showing how to use these concepts in specific equations. This topic includes subtopics on different ways to do just that. This principle is of great help in finding the value of variables. The most important rule of solving equations in algebra is that anything done to one side of an equation must be done to the other.

FOIL Method Used to Distribute Two Binomials.

It then goes on to explain how to put two or more expressions together with arithmetic operations and how to rewrite them in different forms. This topic begins with an introduction to algebra and algebraic expressions. These are mathematical statements that include a variable but no equal sign. The fundamental concepts of algebra revolve around writing algebraic expressions. This guide ends with information about functions. After that, there are explanations of binomials, trinomials, and higher-order polynomials. This page includes resources for all algebra topics, beginning with the basics of algebraic expressions before moving onto solving equations with variables. The techniques used to find the value or range of values of the variable(s) are useful in higher levels of mathematics, including trigonometry and calculus. It is the use of variables that makes algebra distinct from regular arithmetic. The unknown quantity of an algebraic equation is usually represented by a letter, called a variable. They use it when they figure out how much time to budget for a lunch break or decide how many eggs to add to a recipe when making a double batch. While that may sound abstract, most people use algebra every day without realizing it. What is the value of x? The answer to this question usually requires some knowledge of algebra.Īlgebra is a branch of mathematics that involves solving equations and inequalities to find an unknown quantity. So the inequality is satisfied for all values of. Thus the equation has no solutions and since the graph of the function in the left-hand side lies entirely above the -axis. Note that this solution could also be found by applying the special product:įirst we solve the corresponding equation: The graph of the function in the left-hand side is an ‘opens down’ parabola and thus the inequality is only satisfied for: The equation has two coinciding solutions, sometimes it is said that the equation has only one solution. The graph of the function in the left-hand side is an ‘opens up’ parabola and thus the inequality is satisfied by the following values of : In this case we find the solutions of the equation:

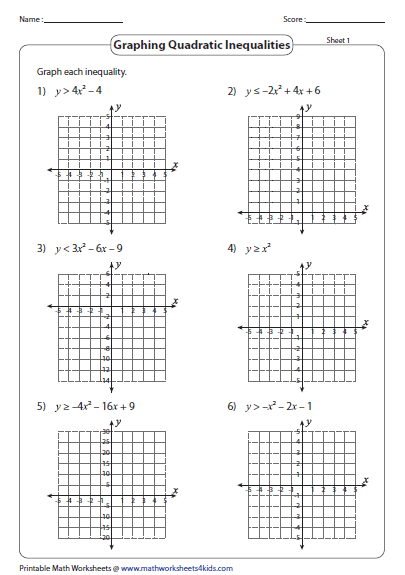
We would have found the same result when applying the -formula.

The parabola ‘opens up’, has two intersection points with the -axis and thus the inequality is satisfied for: We immediately see that the left-hand side can be factorized: The parabola lies entirely above (‘opens up’) or below (‘opens down’) the -axis. In this case the equation has no (real) solutions. For this value of the function equals, for other values of it is greater than (‘opens up’) or less than (‘opens down’). In this case the solutions and are equal. Then, depending on (‘opens up’ or ‘opens down’) we know for which values of the function is greater or less than is. The parabola intersects the -axis in two different points.

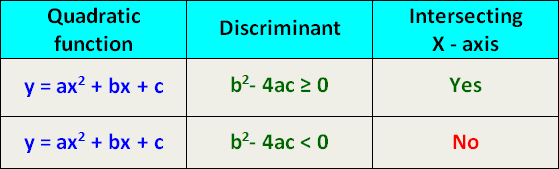
In this case the solutions and are different. Next, we make use of the fact whether the graph of the quadratic function is an ‘opens up’ or an ‘opens down’ parabola. We calculate the solutions of the corresponding equation, and the value of the discriminant: Both methods, factorization or applying the abc-formula, can be used. When solving quadratic inequalities, we must always make use of solving quadratic equations. In Quadratic equations (factorizing) and Quadratic equations (abc-formula) we discussed extensively how this type of equations could be solved. It is advised to study these topics first before you continue with quadratic inequalities. Of course, because otherwise it is no quadratic inequality. A standard quadratic inequality has the same form as the corresponding equation with an inequality sign instead of an equal sign, see the following example: